Trigonometry Questions with Solution for SSC CGL, CHSL, CPO Competitive Exams. Mock Test for free online practice – All type from previous year papers.
Quiz : Trigonometry MCQs
Subject : Mathematics
Important Questions with Solutions
Results
#1. If  and
and  then
then 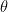 is
is

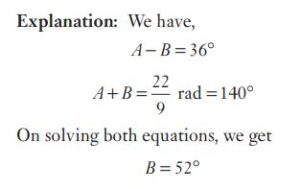
#2. If the sum and difference of two angles are  radian and 36° respectively, then the value of smaller angle in degree taking the value of
radian and 36° respectively, then the value of smaller angle in degree taking the value of 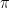 as
as  is.
is.
#3. In circular measure, the value of the angle  15′ is
15′ is

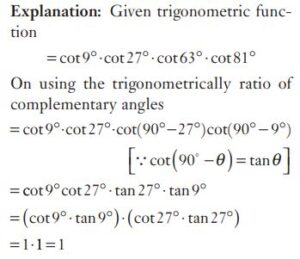
#4. Solve cot  cot
cot  cot
cot  cot
cot 
#5. If tan θ − tan 30° tan 60° and θ is an acute angle, then 2θ is equal to

#6. If $ tanθ + cotθ = 5 $, then tan2θ + cot2θ is:
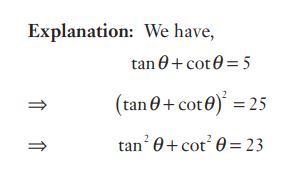
#7. If  = sin2 30 + 4cot2 45 – sec2 60, then the value of
= sin2 30 + 4cot2 45 – sec2 60, then the value of  is:
is:
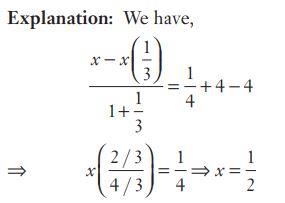
#8. If  , then the value of
, then the value of  is
is

#9. If  , then the value of
, then the value of  is
is
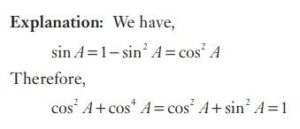
#10. If  and the angle
and the angle 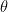 is less than 90°, then the value of
is less than 90°, then the value of 
 is
is
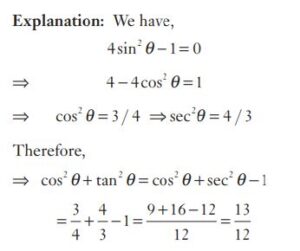
#11. If  , then the value of
, then the value of  will be.
will be.
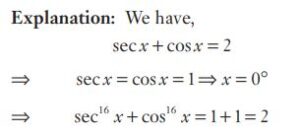
#12. If  , then
, then  is equal to
is equal to
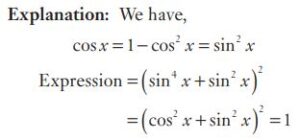
#13. If A is an acute angle and cot A + cosec A = 3, then the value of sin A is :
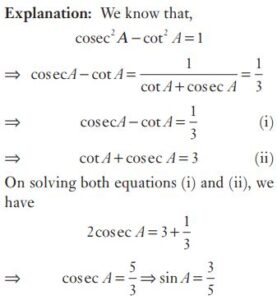
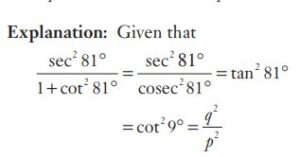
#14. If tan  , then the value of
, then the value of  is
is
#15. If  , then the value of
, then the value of  is equal to
is equal to
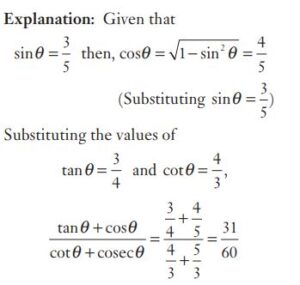
#16. If α and β are positive acute angles, then sin (4α – β) = 1 and cos (2α + β) = 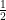 , then the value of sin (α + 2β) is
, then the value of sin (α + 2β) is
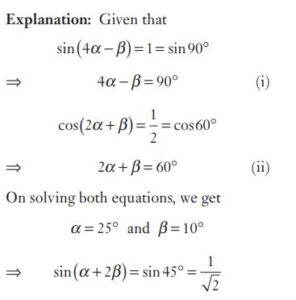
#17. Simplify: 

#18. If 0° < 0 < 90° and 2 sin2θ + 3 cosθ = 3 , then the value of θ is
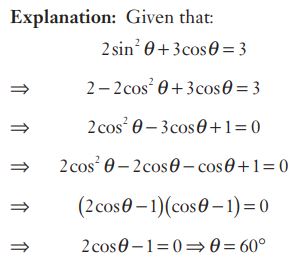
#19. If tan θ +cot θ = 2 , then the value of tan100θ + cot100θ is
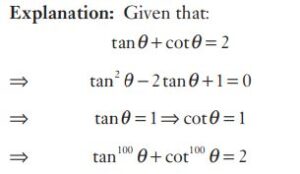
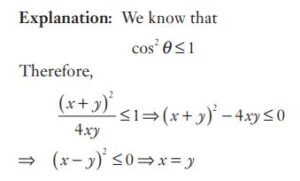
#20. The equation  is only possible when
is only possible when
#21. If cos4θ – sin4θ = 2/3, then the value of 1 – 2 sin2θ is
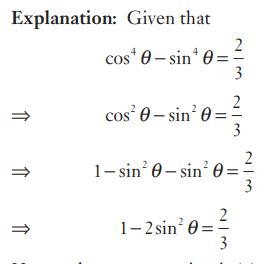
#22. If 7 sin2θ + 3 cos2θ = 4  , then the value of θ is :
, then the value of θ is :

#23. If A = tan 11° tan 29°, B = 2 cot 61° cot 79°, then which of the following is correct?

#24. The measure of the angles of a triangle is in the ratio 2 : 7 : 11. The measures of angle are
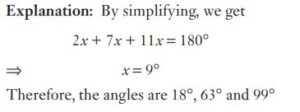
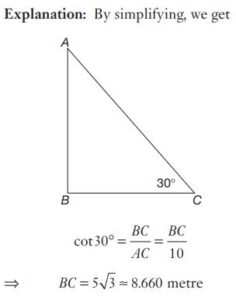
#25. A 10 metre long ladder is placed against a wall. It is inclined at an angle of 30° to the ground. The distance (in m) of the foot of the ladder from the wall is (Given = 
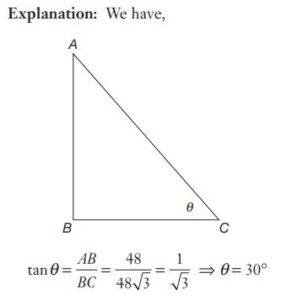
#26. If a 48 m tall building has a shadow of 48  m, then the angle of elevation of the sun is
m, then the angle of elevation of the sun is
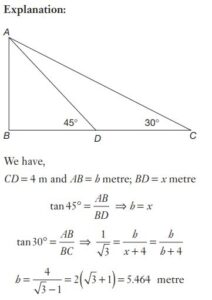
#27. The angle of elevation of sun changes from 30° to 45°, the length of the shadow of a pole decreases by 4 metres, the height of the pole is (Assume  )
)
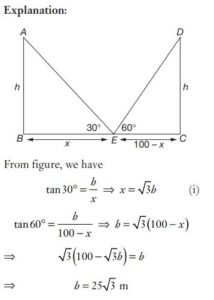
#28. Two poles of equal height are standing opposite to each other on either side of a road which is 100 m wide. From a point between them on road, the angles of elevation of their tops are 30° and 60° The height of each pole (in metre) is
#29. The angle of depression of a point situated at a distance of 70 m from the base of a tower is 60° The height of the tower is :

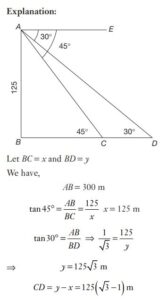
#30. From a tower 125 metres high, the angle of depression of two objects, which are in horizontal line through the base of the tower are 45° and 30° and they are on the same side of the tower. The distance (in metres) between the objects is