Trigonometry Questions with solution for Competitive Exams. Previous year Exam Question with official answer and detail explanation with short tricks. The Questions are as per SSC CGL and CHSL Exam Syllabus and Pattern. The below 1-18 questions are from the all shifts of SSC CHSL Exam.
Trigonometry Questions with Solution
Q.1: If (sin A – cos A) = 0 , then what is the value of cot A ?
(A) $ \frac{\pi}{6}$
(B) 0
(C) 1
(D) $ \frac{\pi}{4}$
(sin A – cos A) = 0
sin A = cos A
$ \frac{cos A}{sin A} = 1$
cot A = 1
Q.2: If $ cosec\theta=\frac{41}{9}$ and $ \theta$ is an acute angle, then the value of $ 5 tan\theta$ will be:
(A) $ \frac 98$
(B) $ \frac {11}{8}$
(C) $ \frac {13}{4}$
(D) $ \frac 78$
$ cosec\theta=\frac{41}{9}$
$ sin\theta=\frac{9}{41}$
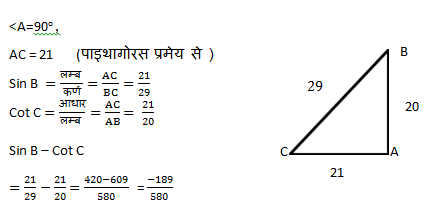
पाइथागोरस प्रमेय से
BC2 = 412 – 92
$ BC =\sqrt{1600} = 40$
ATQ $ 5 tan\theta = 5\times \frac{9}{40}$
$ =\frac98$
Q.3: Solve the following equation and find the value of $ \theta$.
$ 3 cot\theta + tan \theta -2\sqrt3 = 0,0 <\theta < 90^o$
(A) 60o
(B) 30o
(C) 15o
(D) 45o
$ 3 cot\theta + tan \theta -2\sqrt3 = 0$
Put $ \theta = 60$ its satisfy
3 x cot60 + tan60 -2 $ \sqrt{3}$
$ 3\times\frac{1}{\sqrt3} +\sqrt3 – 2\sqrt3$
$ \frac{3}{\sqrt3} +\sqrt3 – 2\sqrt3$
$ \frac{6}{\sqrt3} -2\sqrt3$
$ \frac {6-6}{\sqrt3} = \frac{0}{\sqrt3} = 0$
so $ \theta = 60$
Q.4: In $ \triangle ABC$ , $ \angle A = 90^0$, AB = 20cm and BC = 29cm. What is the value of (sinB -cotC) ?
(A) $ \frac{189}{580}$
(B) $ -\frac {9}{29}$
(C) $ \frac{9}{29}$
(D) $ -\frac{189}{580}$
Q.5: If tanx = cot(48o + 2x), and 0o <x <90o , then what is the value of x ?
(A) 14o
(B 12o
(C) 21o
(D) 16o
tan x = cot (48 + 2x)
tanx = tan (90 – (48 + 2x)
x = 90 -(48 + 2x)
x = 90 – 48 – 2x
3x = 42
x = 14
Q.6: If $ 7 sin^2\theta + 3cos^2\theta = 4,0^o <\theta <90^o$ , then the value of $ \theta$ will be:
(A) 45o
(B) 30o
(C) 60o
(D) 75o
$ 7 sin^2\theta + 3cos^2\theta = 4$
$ 4 sin^2\theta + 3sin^2\theta +3cos^2\theta= 4$
$ 4 sin^2\theta + 3(sin^2 \theta + cos^2\theta)= 4$
$ 4sin^2\theta + 3\times1 = 4$
$ 4sin^2\theta = 4 – 3 = 1$
$ sin^2\theta =\frac 14$
$ sin\theta =\frac 12$
$ \theta = 30^o$
Q.7: Find the value of $ \theta$ , If sec2 $ sec^2\theta +(1 -\sqrt3)tan\theta -(1 +\sqrt3)=0$, where $ \theta$ is an acute angle.
(A) 30o
(B) 15o
(C) 60o
(D) 45o
$ sec^2\theta +(1 -\sqrt3) tan\theta -(1 +\sqrt3) =0$
put $ \theta = 60^o$ (By option)
$ sec^2(60) + (1 -\sqrt3) tan 60 – (1 +\sqrt3)$
$ 4 +(1 – \sqrt3) \sqrt3 – (1 + \sqrt3)$
$ 4 +\sqrt3 – 3 – 1 -\sqrt3$
4 – 4 = 0
So $ \theta = 60$
Q.8: If $ cos\theta =\frac{7}{3\sqrt6}$ and $ \theta$ is an acute angle, then the value of $ 27 sin^2\theta -\frac 32$ is:
(A) 15
(B) 12
(C) 1
(D) 9
Q.9: In a triangle ABC , right-angled at C, if sec A $ =\frac{13}{5}$ , then find the value of $ \frac{1+ sinA}{cosB}$.
(A) $ \frac{18}{5}$
(B) 5
(C) $ \frac32$
(D) $ \frac{25}{12}$
Q.10: If $ sin\theta + cosec \theta = 7$ , then what is the value of $ sin^3\theta + cosec^3\theta$ ?
(A) 322
(B) 382
(C) 367
(D) 350
$ sin\theta + cosec \theta = 7$
$ (sin\theta + cosec \theta)^3 = 7^3$
$ sin^3\theta + cosec^3 \theta +3sin\theta cosec\theta\times7 = 343$
$ sin^3\theta + cosec^3 \theta +3\times7 = 343$ [$ Sin\theta\times Cosec\theta = 1$]
$ sin^3\theta + cosec^3\theta = 343 – 21 = 322 $
Trigonometry Questions for Competitive Exams
Q.11: If 3 cot A = 4 tan A , and A is an acute angle, then what will be the value of Sec A ?
(A) $ \frac{\sqrt7}{2}$
(B) $ \frac{1}{\sqrt3}$
(C) $ \frac12$
(D) $ \frac{\sqrt21}{3}$
Q.12: What is the value of $ \frac{cos^220^o + cos^270^o}{sin^290^o} -tan^245^o$ ?
(A) -1
(B) 0
(C) -2
(D) 1
$ \frac{cos^220^o + cos^270^o}{sin^290^o} -tan^245^o$
$ \frac{1}{1} -1$ [Cos220 + Cos270 = 1, tan 45 = 1]
= 0
Q.13: If $ \sqrt{13} Sin \theta = 2$ , then the value of $ \frac{3tan\theta +\sqrt{13}Sin\theta}{\sqrt{13}Cos \theta-3 tan \theta}$ is:
(A) 5
(B) $ \frac 12$
(C) 3
(D) 4
Q.14: In a right-angled triangle ABC right angled at C, Sin A = Sin B. What is the value of Cos A ?
(A) 1
(B) $ \frac{\sqrt3}{2}$
(C) $ \frac 12$
(D) $ \frac{1}{\sqrt2}$
Sin A = Sin B
A = B = 45 Satisfy
Cos A = Cos 45
= $ \frac{1}{\sqrt2}$
Q.15: If $ 5 Cos \theta = 4 Sin \theta , 0^o \leq \theta \leq 90^o$ , then what will be the value of $ Sec \theta$ ?
(A) $ \frac{\sqrt{41}}{4}$
(B) $ \frac{\sqrt{41}}{16}$
(C) $ \frac{\sqrt{41}}{5}$
(D) $ \frac35$
Q.16: The value of $ \frac{Cos 8^o Cos 24^o Cos 60^o Cos 66^o Cos 82^o}{Sin 82^o Sin 66^o Sin 60^o Sin 8^o Sin 24^o}$ is:
(A) $ \frac{1}{\sqrt2}$
(B) $ \frac{1}{\sqrt3}$
(C) 1
(D) 0
Cos 8o Cos 82o = 1
Cos 24o Cos 60o = 1
Sin 82o Sin 8o = 1
Sin 66o Sin 24o = 1
so $ \frac{Cos 66^o}{Sin 60^o}$
$ Cot 60=\frac{1}{\sqrt3}$
Q.17: If Sin B =$ \frac{9}{41}$ , then what is the value of Cot B, where 0o <B<90o ?
(A) $ \frac{9}{41}$
(B) $ \frac{41}{9}$
(C) $ \frac{9}{40}$
(D) $ \frac{40}{9}$
Q.18: If $ tan\theta =\frac43$ , then the value of $ \frac{9 Sin\theta + 12 Cos \theta}{27 Cos\theta -20sin \theta}$ will be equal to:
(A) 36
(B) 100
(C) 18
(D) 72
Thanks for study the Trigonometry Questions for Competitive Exams in English
Maths Practice Set for Competitive Exams
Number System Questions with Answers
Percentage Questions with Answers
Simple and Compound Interest Questions with Answers
Time and Work Questions with Answers
Mensuration Questions with Answers
Algebra Questions with Answers
Trigonometry Questions with Answers
Pipe and Cistern Questions